Problem #8

It is relatively easy to see that the largest regular tetrahedron which
will fit inside a cube whose edges have length 1 is the tetrahedron shown
in the figure below. The length of an edge of this tetrahedron is Sqrt[2].
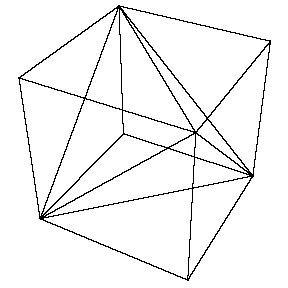
This month there are two questions:
- What is the length of an edge of the largest regular tetrahedron which
will fit inside a regular octahedron whose edges have length 1?"
- What is the length of an edge of the largest cube which will fit
inside a regular tetrahedron whose edges have length 1?"
 |
 |
| tetrahedron |
octahedron |
(GIF's © 1998,
R. Mäder)




