Problem #1

Consider tilings of a 1×n rectangle by a (possibly disconnected) tile consisting of unit squares.

• Suppose all of the tiles must be a translates of one another. For example, there are four such tilings of a 1×4 rectangle as shown below (each tile is colored a single color). The first tiling has four unit tiles, the second has two connected tiles, the third has two disconnected tiles, and the last has a single tile.

  How many such tilings are there of a 1×12 rectangle?

  Source: Harvard-MIT Mathematics Tournament

• Can you determine a formula for the number of such tilings of a 1×n rectangle?

• Suppose we allow the tiles to be translates or reflections of one another. Such a tiling of a 1×6 tile is given below. Tilings that are reflections of one another are to be considered distinct.

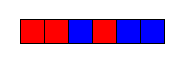
  How many of these tilings are there of a 1×12 rectangle?

• Can you determine a formula for the number of these tilings of a 1×n rectangle?

The solution will be posted shortly.

Back to the Archives